If interested in purchasing my NEW 2012 Math Challenge Problem/Quiz book, click on BUY NOW at top of right sidebar. 175 problems divided into 35 quizzes with answers at back. Suitable for SAT/Math Contest/Math I/II Subject Tests and Daily/Weekly Problems of the Day. Includes both multiple choice and constructed response items.
Price is $9.95. Secured pdf will be emailed when purchase is verified. DON'T FORGET TO SEND ME AN EMAIL (dmarain "at gmail dot com") FIRST SO THAT I CAN SEND THE ATTACHMENT!
------------------------------------------------------------
Update2: See the awesome article in MathWorld on tangential quadrilaterals for more info re problem #2.
Update: See Comments section for some answers, solutions.
Part (a) of each of the following are somewhat difficult questions that can be found in some geometry textbooks. These are numerical exercises and good practice for the more difficult SAT-types of questions or for math contests. The last part of each question is an extension or generalization of the problem. Texts do not often ask students to delve beneath the surface and look for general relationships.
1. A circle of radius 4 is inscribed in a right triangle with hypotenuse 20.
(a) Find the perimeter of the triangle without using the Pythagorean Theorem. Justify your reasoning.
(b) Using the Pythagorean Theorem, show that the triangle is similar to a 3-4-5 triangle.
Note: Many students tend to guess multiples of 3-4-5 when doing these. Sometimes they get lucky but they need to prove it!(c) PROVE in general that the perimeter of a right triangle is twice the sum of its hypotenuse and the radius of its inscribed circle. Again, no Pythagorean Thm allowed.
Note: There are well-established formulas for the inradius of a triangle. Our objective here is to look at one special case.
2. A circle is inscribed in a quadrilateral which has a pair of opposite sides equal to 12 and 18. Neither pair of opposite sides of the quadrilateral is parallel.
(a) Find the perimeter of the quadrilateral. Justify your reasoning.
(b) PROVE in general that the perimeter of a quadrilateral in which a circle is inscribed equals twice the sum of either pair of opposite sides.
Note:: Not all quadrilaterals have an inscribed circle, so this is a strong condition.
Note: As always, these results need independent verification. I welcome your comments and edits!
Friday, March 30, 2007
Challenging Geometry: Circles Inscribed in Quadrilaterals, Right Triangles
Posted by
Dave Marain
at
6:12 PM
15
comments
![]()
![]()
Labels: circles, geometry, inscribed, quadrilaterals, SAT-type problems, tangents
Monday, March 26, 2007
Lattice Points Problem Part 2: Circles, Gauss' Circle Problem and Pick's Theorem
Eric Jablow inspired me to develop the following extended enrichment actvity/project for Geometry students. You can research the general solution of Gauss' Circle Problem in MathWorld but for this application it isn't necessary to use that formula.
Consider the circle of radius 5 centered at the origin.
(a) Determine the coordinates of the 12 lattice points on this circle. Recall that lattice points are points whose coordinates are both integers.
Note: Is it reasonable from symmetry arguments that the number of lattice points is divisible by 4?
(b) Using graph paper, show there are 69 lattice points INSIDE this circle. Describe your counting method.
The total number of lattice points inside or on our circle is 69+12 = 81. This agrees with the result from Gauss' formula but we will now 'approximate' this result using Pick's Theorem which gives the relationship among interior, boundary points and the area of a polygon whose vertices are lattice points.
(c) Consider the inscribed dodecagon formed by connecting the 12 lattice points from part (a). Determine the lengths of the sides of this polygon.
(d) By dividing the polygon into 12 triangles show that the area of this polygon is 74. No trigonometry, just Pythagorean and basics!
Hint: This is not a regular polygon but you can still divide it into 12 isosceles triangles.
Comment: Do you find it surprising that the area is rational (in fact, integral), considering that the sides are irrational?
(e) Pick's Theorem states that the area of a polygon whose vertices are lattice points is given by the formula A = I + B/2 - 1 where I = the number of interior lattice points and B = the number of boundary lattice points, that is, points on the polygon.
Show that Pick's Theorem leads to I = 69.
(f) For our problem the number of lattice points inside the circle matched the number of points inside the inscribed polygon. A coincidence? Whether it's true or not, explain why this result seems to make sense.
(g) To further investigate this 'coincidence', change the radius to 10.
(i) Show, by counting, that there are 317 lattice points inside or on this circle.
(ii) Show that there are still 12 lattice points on this larger circle.
(iii) Show that there are 303 lattice points inside or on the resulting dodecagon.
[As before, find the area w/o trig and use Pick's Thm. Note: To find the area of the polygon use (d) and ratios!]
(iv) Show that the point (4,9) is inside this circle but outside the dodecagon! This suggests why Pick's theorem fails in this case! Why?
Good luck! This is an extended challenge that I will leave up for several days and invite comment. Whether you implement it in a classroom or not, enjoy!
[By the way, some of the numerical results (like 303) have not been independently verified. If you find an error, let me know!]
Posted by
Dave Marain
at
6:55 PM
1 comments
![]()
![]()
Labels: area, circles, dodecagon, Gauss Circle Problem, geometry, lattice points, Pick's Theorem
Sunday, March 25, 2007
In-Depth Investigation of Patterns: Lattice Points, Algebra,...
Update!
Answers to General Formulas for |x| + |y| = N:
ON: 4N
INSIDE or ON: N2 + (N+1)2 = 2N(N+1) + 1 = 1+4+8+...+4N
OUTSIDE the 'diamond' but INSIDE or ON surrounding square:
2N(N+1) = 4+8+...+4N
TOTAL: (2N+1)2
[Pls indicate any discrepancies you find!]
The following starts with a fairly simple pattern but watch out -- students from Middle School through Algebra 2 and beyond can take this as far as their imagination and skill can carry them. The questions below review absolute values, inequalities, graphs, geometry, etc., but that is the tip of the iceberg. Prealgebra students should start with simpler values to get the idea. Have them make a table of their findings as suggested at the bottom.
One could begin with a variation on an SAT-I, SAT-II type or math contest question:
How many ordered pairs (x,y), where x and y are both integers,
satisfy |x| +|y| ≤ 6?
Note: If this were to appear on the 'new' SAT, the inequality would be replaced by an equality. or the '6' would be replaced by a smaller number.
Here's a restatement using the terminology of lattice points:
In the coordinate plane, a point P(x,y) is said to be lattice point if both coordinates are integers.
How many lattice points are inside or on the graph of |x| + |y| = 6?
Answer: 85
Sorry for giving it away, but the objective is to discover ways to derive this result, not the result itself.
When I see questions like this, my inclination (as a mathematician) is to generalize, that is, try to understand a general relationship if the '6' were replaced by N.
Here is the more advanced generalization (one could go further!):
We are investigating the number of lattice points inside or on the graph, G, of
|x| + |y| = N, where N is a positive integer. We will also consider the smallest square, S, whose sides are parallel to the coordinate axes in which the graph of
|x| + |y| = N is inscribed. [This really needs to be drawn but I'll leave it to the reader's imagination. The top side of this square is part of the line y = N.]
(a) Derive a formula for the number of lattice points inside or on G.
(b) Derive a formula for the number of lattice points inside or on S
(c) Derive a formula for the number of lattice points outside G but inside or on S.
Notes, Comments:
For the younger students, or for any group that isn't quite ready for the above, I strongly recommend building the pattern one step at a time and to record their results in a table:
|x| + |y| = 0 (o is not a positive integer but it makes sense to start here) to |x| + |y| = 1 to
|x| + |y| = 2,...
Also, there are alternate formulations (or a variety of patterns) for the general number of lattice points. Don't be surprised if your students find them! Of course, they should be encouraged to show they are all equivalent. Questions about the geometry of the figures could also be raised (if time permits). For example, some students do not fully appreciate that the diamond-shaped graph of |x| +|y| = N is in fact a square, Now, what would its area and perimeter be...
This investigation can be started in class then assigned to be finished outside of class, preferably with a partner. I plan on starting this with my 9th graders on Mon or Tue but I will need to review absolute values, graphs, etc., and I will develop it incrementally. I will be more than happy if, in one 40 minute period, they can complete a table showing the correct number of lattice points for N = 0,1,2,3!
[Additional but critical point: I am not suggesting that these kinds of extensive explorations should ever replace the need to deliver content and develop skills. These are intended to be enrichment activities, no more, no less!]
Posted by
Dave Marain
at
6:11 AM
8
comments
![]()
![]()
Labels: advanced algebra, algebra 2, geometry, investigations, lattice points, open-ended, patterns, prealgebra
Saturday, March 24, 2007
Carnival of Mathematics Selection
I just want to thank Prof. Jason Rosenhouse for selecting MathNotations for the March 23rd edition of the Carnival of Mathematics. Click on the link in the sidebar to see many other excellent math blogs selected by Jason as well as links to earlier Carnivals. This sidebar is continually updated - enjoy the many other outstanding math blogs linked there.
Here is Jason's description of two of the posts you may have recently read:Dave Marain of MathNotations has a fascinating post on the evolution of a difficult standardized test math problem. Math and evolution? My kind of post! See also his post on Geometry and Reasoning. Much food for thought.
Posted by
Dave Marain
at
6:25 AM
0
comments
![]()
![]()
Labels: carnival of mathematics
Thursday, March 22, 2007
Daniel E. Richman - Recipient of DOE 2007 National Undergraduate Fellowship Award
It is my great pleasure to announce that Daniel, one of my former students, has been selected to be a recipient of the 2007 National Undergraduate Fellowship Program for summer 2007.
Daniel was an outstanding student in my BC Calculus class 3 years ago. It was obvious to me then that he was unique among his very talented peers. Even then I recognized that he was a deep conceptual thinker who wanted to understand the why as much as the how. His maturity of mind was extraordinary. Further he is a profoundly moral young man of outstanding character. Daniel is a junior at the University of Rochester. Congratulations, Daniel, to you and your wonderful family.
The following are excerpts from the article on the website.
The National Undergraduate Fellowship Program in Plasma Physics and Fusion Energy Sciences provides outstanding undergraduates with an opportunity to conduct research in the disciplines that comprise the plasma sciences in general and fusion research in particular.
The program is intended primarily for students completing their junior year majoring in physics or engineering, but highly motivated students completing their sophomore year are encouraged to apply as well. The nine-week long research projects are performed at one of the many participating universities and national laboratories throughout the country. The goal of the Program is to stimulate students' interest in the fields relevant to fusion research while providing capable assistants for fusion research projects. In order that the students obtain a sufficient background to begin their research projects, the nine week project is preceded by a one week introductory course at the Princeton Plasma Physics Laboratory in the basic elements of plasma physics, after which the students travel to the sites of their research projects.
The Program is funded by the U.S. Department of Energy, Office of Fusion Energy Sciences.
Both Sam and Daniel were selected from the "largest number of applicants in the program's history", according to James Morgan, NUF Program Leader. Sam will be joined by other awarded recipients from around the country.
Daniel will spend the summer at the MIT Plasma Science & Fusion Center. The experience also includes spending the first week of the summer program at Princeton University taking a course in introductory plasma physics.
Daniel E. Richman,class of 2008,is studying towards a BS in Physics, a BA in Mathematics and a BA in Music.
Posted by
Dave Marain
at
1:28 PM
0
comments
![]()
![]()
Wednesday, March 21, 2007
Searching continued...Another Challenging Combinatorial Problem
The 'answers' to the problems below are now posted in the comments...
[Update: I modified today's problems for my 9th grade group. An excerpt from the handout is shown at the bottom. How do you think they did? These are youngsters who need very precise instructions and find math more challenging. Guess how they did?]
How many 4-digit numbers have exactly 2 identical digits?
Today's questions were inspired by a Google search from one of the viewers of this blog. No matter how many of these types our students may attempt, there is no substitute for a systematic approach and clear thinking. Professional mathematicians who have done numerous problems of this type and are therefore likely to know an efficient method still can make careless logic errors. Imagine how our students do! They're looking for a quick and dirty approach, one short-cut method or formula that covers all kinds. Not likely!!
There's an interesting semantics problem with this question: Does 3232 count as a possible answer? Your first instinct might be to say, "Of course, it doesn't count!" But how many identical digits does it really have? For this reason, I will reword today's question to:
How many 4-digit positive integers have exactly one pair of identical digits?
[Note: Someone will probably argue about the semantics here as well but I'll let it stand!]
I'll post the result I obtained later but, for now, how many different approaches do you think your students could come up with? I thought of at least 3 not to mention writing a short program to count them!]
I see this kind of problem as appropriate for middle school and high school. For the middle grades, students should begin with the 3-digit version (see worksheet below) and be encouraged to make an organized list. By high school, they should be able to move on to more powerful counting methods but we know some are stuck at the 4th grade level of counting in an unorganized manner. In fact, from my observations, most high schoolers start in the 1000's, count those and multiply by 9. This is actually a fine method but should they know other approaches by the time they complete Algebra 2?
The following is a portion of the worksheet I gave to my group today. It worked out well. Any thoughts? Notice that I modified the 4-digit problem to make it more accessible for them. It provided an easier extension.
-------------------------------------------------------------------------------------
Posted by
Dave Marain
at
5:37 AM
8
comments
![]()
![]()
Labels: combinatorial math, digit problems, SAT-type problems
Tuesday, March 20, 2007
Searching...
Based on a reading of Google searches of visitors to this site, here are a few of the more common topics, rephrased as questions, that I have noticed. I also noted that, aside from pi day, which generated hundreds of visits from those looking for historical information about pi and the names of mathematicians, many are looking for sample MathCounts problems or references to books of these.
1. What is the largest 3-digit prime with 3 prime digits?
Ans: 773 if repeated digits are allowed; 523 if not. This question has appeared in one of my earlier posts. Must be a fairly common one that readers come across from math contests or from class. A quick list of primes up to 1000 can be found at VIAS Encyclopedia.
2. How are the following questions related:
How many different handshakes occur if each of 10 people shakes hands with each of the remaining people in a room? (can be expressed more clearly)
How many different segments can be formed by connecting the vertices of a decagon in all possible ways? (can also be expressed in terms of the number of chords formed by 10 points on a circle)
Ans: Both problems can be expressed as 9+8+7+6+...+2+1 or (9)(10)/2 or 10C2, the number of combinations of 10 objects chosen 2 at a time. In general, for n people or points on a circle: 1+2+3+4+...+(n-1) = (n-1)(n)/2 = nC2. The equivalence comes from the fact that each handshake or each chord is uniquely determined by selecting 2 people or 2 points. To avoid repetition we use combinations rather than permutations.
4. How many different seven-digit phone numbers (ignoring the area code) can be formed?
Ans: If any digit 0-9 were allowed in all positions then there would be 107 possibilities since there are 10 choices for each digit (using the Multiplication Principle here). Subject to restrictions on zeros or other digits or other local considerations there would be fewer.
Here's a related question that I have not yet seen:
How many different IP addresses are possible worldwide if every computer, device etc., must have a unique one?
I believe that IP addresses are always of the form xxx.xxx.xxx where the first digit in each group is allowed to be zero, that is, one could use a 2-digit number in each group. For example, 66.19.35 is acceptable. I didn't research the rules so this may be incorrect.
Ans: If the rule of formation is accurate, there could be 109 or one billion IP addresses. How long will it take for these to be used up? I know someone out there will tell us how this is or will be handled!
Update on IP Addresses: My belief about the form of IP addresses was dead wrong! The protocol should have been 4 groups of integers, each in the range from 0 through 255. There are now newer protocols to allow for the exponential growth of devices needing an address. See the comments for this post to learn more from those far more knowledgeable than myself!
Posted by
Dave Marain
at
5:35 AM
3
comments
![]()
![]()
Labels: combinatorial math
Monday, March 19, 2007
Developing Algebraic Reasoning
The following sequence of problems deals with a fairly well-known pattern. Similar questions have appeared on SATs, on other standardized tests and in texts. The intent here is to provide an extended activity for students of diverse math backgrounds and abilities to develop a systematic approach to analyzing patterns. Students should also be encouraged to make a table of values in which the first column is the number of 'crosses' and remaining columns are reserved for other 'dependent' variables. This function-based approach is also an essential feature of this development.
Notes: There are many ways to approach these questions. Encourage students to share theirs! These questions involve pattern-based thinking, combinatorics, recursive sequences, arithmetic sequences and algebraic reasoning. Parts (d) and (e) are more challenging for some. Based on the pattern of the first 3 or 4 terms, some students will simply develop a linear formula of the form aN+b for the perimeter (which is somewhat harder than the area). It is important for our prealgebra and algebra students to recognize that any arithmetic sequence like 12,20,28,36,… can be described this way. My experience is that if a class has 20 students, there will be at least 5 different ‘counting’ methods discussed, Students often are very creative here and not all use ‘linear’ thinking!
Posted by
Dave Marain
at
7:21 AM
0
comments
![]()
![]()
Labels: algebra, linear function, patterns, sequences
Saturday, March 17, 2007
The Genius of Archimedes: Parabolas, Tangents...
Pi day is over, but it seems fitting to continue exploring. Archimedes did more than develop an approximation procedure for pi! There are many excellent websites that explain the following in greater detail and discuss many more of Archimedes' theorems about parabolas and tangents. I attempted to draw a diagram using Draw in Word. It's crude but you'll get the idea. The object is to share this extraordinary piece of history of mathematics and have your students finish the proof that a light ray from the focus that strikes a parabolic surface is reflected in a ray that is parallel to the axis of the parabola. This is equally interesting in reverse: External light rays and other forms of electromagnetic radiation that are parallel to a parabola's axis are reflected to the focus, very useful for radar and other 'collection' devices.
Considering that Archimedes' proofs used only geometric properties makes his work even more astounding (now of course we can use coordinate geometry, calculus, etc.). This type of investigation is usually deferred to College Geometry courses, but I believe we can deliver it to motivated geometry, 2nd year algebra or precalculus students. If nothing else, it makes for a wonderful long-term project!
Ok, here goes...
In the diagram below, I've gone out of my way to make the reflecting ray NOT look parallel to the axis, even though we're trying to prove it is. This is to help students avoid assuming collinearity, when, in fact, that needs to be proved!
The two angles marked X are equal by a reflection principle (angle of incidence equals...). The two angles marked Y are equal because it can be proved that the tangent line at P is the perpendicular bisector of segment FP', where F is the focus and P' is the foot of the perpendicular from P to the directrix. I chose not to derive Archimedes' very subtle argument, but it is worth studying the proof. The proof starts by constructing the perpendicular bisector and showing that this line passes through P but no other point of the parabola, thus it is tangent. Alex Bogomolny's excellent and in-depth treatment (with java applets) of this topic (on cut-the-knot) is very worthwhile reading.
The student is being asked to prove that the reflecting ray is parallel to the axis. This is equivalent to showing that the line containing PP' and the reflecting ray are one and the same. The argument is straightforward, but students may want to continue learning more about the genius of Archimedes.
[Good luck copying this diagram (jpg). Some of you may find errors in my argument or an extremely simply argument for the parallelism, so pls share!!]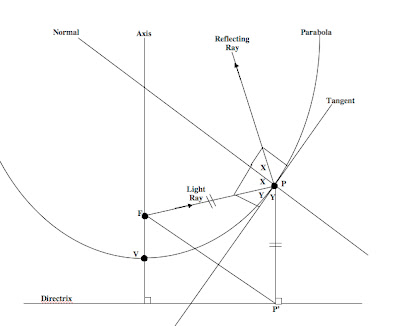
Posted by
Dave Marain
at
10:02 AM
0
comments
![]()
![]()
Labels: archimedes, geometry, logic, parabolas, pi, proof, tangents
Thursday, March 15, 2007
Advanced Algebra Challenge
Note: Many of the ideas below came from the excellent note in the Reader Reflections of the March 2007 Mathematics Teacher, contributed by Warren Groskreutz. One of his students derived and proved his own 'theorem' about certain radical expressions. I decided to develop these ideas into an activity. The last part is a bit different from 'Nathan's Theorem.' Mr. Groskreutz is to be commended for creating an environment in which such 'discoveries' can be made.
[Click on the small image below to enlarge.]
Posted by
Dave Marain
at
6:51 AM
0
comments
![]()
![]()
Labels: advanced algebra, radicals
Wednesday, March 14, 2007
A Collection of Pi Poems...
Here are some Pi Poems from our students. They’re not ready for publication yet, but our teachers enjoyed these. I’ve removed their names of course. If you have student work you’d like to share, pls do so!
See Eric Jablow's comment on previous post for a very clever one!
How I love a sweet chocolate pi Sunday after noon =
3.141592654
It's a pain I wasn't competent at ratios, sines, and
stuff.
Now I want a puppy, dalmation, or poodle that's cute.
How I love a tasty delicious pi! Eating apple pie feels
dynamite, fantastic, perfect, excellent, and so fun! =
3.14159265358979323
Now I know a great geologist on planet Earth.
How I wish I could regularly be around great math!
This one unfortunately has an error in one of
the digits but I thought it was a really great effort
nonetheless...
Pie I like a peach blueberry or banana cream and lemon
meringue rasberry rhubarb mincemeat pie in sky apple ala
mode cherry or humble mud or pumpkin chocolate pecan oh
ruin your appetite
Posted by
Dave Marain
at
10:51 AM
0
comments
![]()
![]()
Tuesday, March 13, 2007
Wed 3-14 A Pi-Fect Day!
3.141592653589793238462643383279502884197169399375105820
97494459230781640...
Don't forget to celebrate Pi Day on 3-14-07 at 1:59 PM!! Considering how many out there are searching for information about Pi, this has become a huge event. Although we may not be quite ready for an official national holiday, many now refer to 3-14 as National Pi Day!
Here are three of the best Pi-Links I have found. You may want to visit them to learn more about one of mathematics most fascinating numbers - more than you may ever have wanted to know! The Wikipedia Pi article is also wonderful.
Exploratorium
A History of Pi
The Pi-Search Page
Enjoy the day but don't forget that in many other countries, Pi Day is celebrated on July 22nd in honor of the approximation 22/7! Personally, I've always been 'partial' to 355/113.
Posted by
Dave Marain
at
7:54 PM
1 comments
![]()
![]()
Saturday, March 10, 2007
Parabolas, SATs, Quadratic Functions, Symmetry, Oh My!
The new SAT and other state math assessments are or will be including more Algebra 2 types of questions, particularly those involving quadratic functions. The following was inspired by a recent SAT math problem. As usual, my goal here is not to give conundrums and 'puzzlers'. I'll leave that to the expertise of Jonathan over at jd2718! My intent is to provide enrichment and extensions of questions that students are doing in class. More time is required for these than is normally given for an example presented by the teacher. Hopefully these can be used in the classroom.
The original question on the SAT gave a particular length for segment PQ (see below) and that may be a more reasonable start for most Algebra 2 students. The objective here is to have students apply and extend their knowledge of quadratic functions, graphs, coordinates, symmetry, etc. There are several approaches to this question. If instruction enables students to investigate this problem for 10-15 minutes, students may discover alternate methods that will deepen their understanding of the material. The teacher's role is to gauge the ability level and background of the group to determine how much structure/guidance is needed. This is not obvious at all and requires considerable pedagogical skill and experience.
Consider the graph of the quadratic function f(x) = x2. Assume P, Q are points on the graph so that segment PQ is parallel to the x-axis and let the length of segment PQ be denoted by 2k.
If the graph of g(x) = b - x2, intersects the graph of f(x) at P and Q, express the value of b in terms of k.
Notes:
Encourage several methods, i.e., pair students and require that they find at least two different methods. This is critical to develop that quick thinker who always has the answer before anyone else and does not want to deepen his/her insight. Many students will need to start with a numerical value for the length of segment PQ, say 4. Symmetry is a key idea in this problem, not only with respect to the y-axis, but also with respect to segment PQ! Some will see this quickly, others won't. It is our obligation to think this through in advance and be prepared to guide the investigation. Those who believe this kind of activity is a waste of precious time (so much more content could be covered) will never understand why I believe 'less is more' when it comes to learning math. Profound understanding can never be rushed. Short-cuts, IMO, are PART of a discussion, not the objective. Try it! Can you find at least THREE ways?
Posted by
Dave Marain
at
8:04 AM
3
comments
![]()
![]()
Labels: algebra, algebra 2, parabolas, quadratic function, SAT-type problems, symmetry
Friday, March 9, 2007
A Pi Day Scavenger Hunt
If interested in purchasing my NEW 2012 Math Challenge Problem/Quiz book, click on BUY NOW at top of right sidebar. 175 problems divided into 35 quizzes with answers at back. Suitable for SAT/Math Contest/Math I/II Subject Tests and Daily/Weekly Problems of the Day. Includes multiple choice, I/II/III case type and constructed response items.
Price is $9.95. Secured pdf will be emailed when purchase is verified. DON'T FORGET TO SEND ME AN EMAIL (dmarain "at gmail dot com") FIRST SO THAT I CAN SEND THE ATTACHMENT!
------------------------------------------
Note: This link will get you to all of the Pi Day posts/activities on MathNotations.
Here's an idea from one of the outstanding teachers in my department. We will be implementing it on Pi Day this year. Students have been notified in their math classes and on the PA of the following:
On 3-14, there will be questions about various historical decimal approximations to pi posted around the building. Students will have to find these questions, determine the answers and submit their results by the end of the day. In case of many ties, there will be a drawing to determine who wins the free Pizza Pi certificates! We will raise money for the prizes by baking pies and selling them on Mon and Tue in our cafeteria. We are using a wonderful book about Pi for our source of these approximations but I won't mention it yet in case some of our students are visiting this blog (I don't keep it a secret!). I might be slightly off in some of the details but you got the idea.
I've noted that many visitors to this blog are searching for engaging pi day activities. There are many out there already but educators are always looking for fresh ideas. It would be great if you could share these...
By the way, the Pi Activity worksheet I posted yesterday worked fairly well. Once the students got into it, they seemed to enjoy it. Read the comments regarding how they searched for the mathematicians whose birthdays were given. Interesting stuff regarding how some students know how to use 'refined search' techniques in Google but many don't.
Posted by
Dave Marain
at
5:06 PM
0
comments
![]()
![]()
Labels: pi day
Thursday, March 8, 2007
Pi Day Activity Part Two

It may not display well but I'm posting an image of the second part of the Pi activity I posted the other day. This kept the group engaged for over a period. It may not help them to understand the deep meaning of pi or its relation to a circle but they definitely got a sense of the decimal representation of pi and struggled with some of the birthdates, since a simple Google search will not suffice. Do they now appreciate pi more? How will I assess the learning from this? I hope you will see that this was more than just meaningless busywork to keep them quiet! See if you can find all of the mathematicians in less than 10 minutes!
Posted by
Dave Marain
at
10:27 AM
3
comments
![]()
![]()
Labels: mathematicians, pi, pi day
Anatomy and Evolution of a Difficult Math Problem
Note: The problem below was chosen for the March 23rd Carnival of Mathematics. If you'd like to see a different kind of mathematical challenge, I recommend you also visit the posting for 3-30-07 (Challenging Geometry: Circles Inscribed in Quadrilaterals, Right Triangles).
Ever wonder how much thought goes into developing a challenging math question for a standardized test like SATs? The goal on most standardized tests of reasoning is to have a small percentage of questions (usually at the end of a section) that are more discriminating. A question accomplishes this goal if less than, say, one-third of the test-takers answer the item correctly (testing and measurement experts may have a different percent in mind here). The question is first field-tested and if the p-value (% who get it right) is too low, the question may be rejected.
Let's look at how such a challenge problem may evolve.
First Version:
322 has two identical digits. What is the next larger 3-digit number of this type?
Comments: Way too easy since the answer is the next integer, 323 and even students who are not thinking will probably not choose 333 which has 3 identical digits (333 could be 'of this type' since the wording was vague). Some may choose 344 however. Does this question have promise? Can we improve the wording and ratchet it up?
Second version:
6,333 has exactly 3 identical digits. What is the next larger such integer?
Comments: Better? We added 'exactly' to make it clear that it couldn't have more than 3 identical digits, an important distinction. I dropped the 'number of digits' info since it wasn't needed. Will many students choose 6,444 rather than 6,366? Probably, so should we stop here and field test it?
Third version:
81,111 has exactly four identical digits. What is the next larger such integer?
Comments: This should be slightly more discriminating than the previous because the string of ones is more seductive and will probably lead many to be lured into 82,222, rather than the correct answer of 81,888. The stronger student will be suspicious here, particularly if this question is placed near the end of a section. Further, this question cannot appear on the SAT since one cannot grid in a 5-digit answer (4 is the maximum). So we need one more version...
Fourth and final version:
96,666 has exactly four identical digits. If N represents the next larger such integer, what is the value of N - 96,666?
Comments: Ok, now the answer 'fits' in the grid. Experienced item writers and test constructors know that a majority of random test takers will grid in 1111 as the answer, particularly if this is near the end of a section and time is a factor. The student who has stronger reasoning AND can think under pressure will probably realize that N could be 96,999 so the correct answer is 333. Sorry to give it away, but today's post is about constructing a more difficult problem rather than challenging you! BTW, a question very similar to this has appeared on the SATs and in some SAT prep books.
So what's the point of all this and how do I or any item writer know what makes a question harder? EXPERIENCE! (I almost yelled 'TRADITION' since I watched my 13 year old perform in 'Fiddler' last week!). I've given this question or a similar version to SAT prep groups for some time now. In fact, I did it this past Saturday. The results? First group, out of 23 students, 3 answered it correctly. Second group, out of 11, ONE answered it correctly. Since the percentages were considerably less than 20%, would this question ever make it out of field testing? Well, I believe it did! From my own experience, less than 15% of students answer it correctly and my sample space consists of above-average students, in fact, fairly strong students.
How many of you are thinking that this question is unnecessarily 'tricky' and really has no place on a math test? What important math skill or concept is it assessing? Well, some questions are assessing reasoning ability and this is one of them. Does this question fairly discriminate? If the highest-scoring students got it wrong, then it DOES NOT discriminate! However, that's not what happened. 'K' was the only student who answered it correctly in my second group and I predicted she would. She has exceptional reasoning aiblity. She gave me a look of "This wasn't that hard, Mr. Marain, why are you complimenting me so much!" For her it wasn't that hard and that's the point. Is there a place for these kinds of questions on assessments when most students have little exposure to these. Well, the test is not intended to be just a reflection of homework problems from a textbook. This is precisely why many educators and leaders have challenged the SATs over the past few years and why the test was changed a couple of years ago. But there will always be a couple of these...
I'm sure many of you have strong opinions about the educational validity of this question, particularly for a standardized test. If nothing else, try it out in your classes (or give it to your spouse, child, colleague or co-worker) and report the results. If over 50% of the group answer it correctly (give them 30 seconds), I would guess you have one special group there! So, folks, does this much thinking really go into writing one little old test question!
Posted by
Dave Marain
at
4:57 AM
![]()
![]()
Labels: logic, reasoning, SAT-type problems, test construction
Wednesday, March 7, 2007
Update on National Math Panel
As promised, I am posting, with the permission of the National Math Panel, the reply to my latest email to the Panel. Jennifer Graban sent me this last Friday and you can read it for yourself and decide if you believe that some of my recommendations and those of others are now being considered seriously. Below, I will also briefly discuss the conversation I had with Ms. Graban when I called her:
Dear Mr. Marain,
We apologize for the delay in responding to your e-mail of February 11 and acknowledge your ongoing concerns about the composition of the Panel. Understanding the experiences of teachers, particularly algebra teachers currently teaching in the classroom, is important to the Panel and its final report. I have shared your concern with the Panel Chair, Larry Faulkner, as well as other Panel members. We are currently considering additional opportunities that will involve teachers to obtain their vital input in the work of the Panel.
Please know that Panel is most concerned with and plans to carefully consider the perspectives of today's math teachers.
Once again, thank you for your interest in the National Math Panel.
Sincerely,
Jennifer
Jennifer Graban
National Math Panel Staff
U.S. Department of Education
400 Maryland Avenue, SW
Washington, DC 20202-1200
I decided to call Ms. Graban last Friday afternoon around 4 PM EST. She picked up the phone immediately and we spoke for about 10-15 minutes. To the best of my recollection here's how it went:
I began by saying that I felt it important to put a voice to all the emails and blogs and let her know that I am a real person and not the enemy, just someone who feels that not having at least one secondary teacher on this panel was a gross oversight. Continuing, I indicated that, although it would be better to appoint 2-3 dozen classroom math teachers immediately, the reality is that the panel has less than one year to complete its task and publish its final report. Therefore I suggested the importance of identifying a cross-section of a few current high school math teachers with broad experience in both urban and suburban/rural school settings. I also volunteered to provide whatever input I could to the Panel, but certainly this group of teachers should be allowed to serve in an advisory capacity, i.e., freely provide their ideas and be be able to review and make suggestions now and to the final draft of the report before its release. I expressed that this would significantly enhance the credibility of the report. She replied that this was already being considered and she seemed to concur with some of my statements. I also mentioned my call for a national math curriculum but she indicated that the panel has not yet endorsed this. I also asked her if she had noted that the textbook publishers, who were allowed to give extensive presentations to the Panel, had indicated they now had editions for EACH state or nearly so. I expressed how absurd I felt this was and she did not disagree! We left the conversation cordially.
Posted by
Dave Marain
at
6:01 AM
0
comments
![]()
![]()
Monday, March 5, 2007
Problems 3-5 thru 3-6-07: Geometry and Reasoning
Note: The problem below was chosen for the March 23rd Carnival of Mathematics. If you'd like to see a different kind of mathematical challenge, I recommend you also visit the posting for 3-30-07 (Challenging Geometry: Circles Inscribed in Quadrilaterals, Right Triangles).
Today's questions involve well-known ideas from geometry. Similar questions have appeared on SATs and math contests.
Some suggestions: Use it for in-class enrichment or assign it for extra credit outside of class. The first part lends itelf to a fairly simply visual approach (cutting up the square and matching the pieces), but the second is more sophisticated. Encourage the visualization but require the analytical approach as well!
Reviews: 30-60-90, equilateral, areas, symmetry, etc.
Posted by
Dave Marain
at
1:40 PM
7
comments
![]()
![]()
Sunday, March 4, 2007
National Math Curriculum UK
Just to see how England is handling a national curriculum (for all subjects), here's a link to the Mathematics section. You will need to navigate this site for awhile to get the feel for it. You will also want to download the 90+ page pdf math curriculum document. I've skimmed it and it is fascinating. Of course much of it is not new or surprising or that different from NCTM's many recommendations or reports, but it's the overall structure and expectations for the four key stages of learning that make it unique. Why can't we benefit from something like this so that we don't have to reinvent the wheel? I'm certain that members of the National Math Panel are familiar with this but I'd be interested in their views. Is it possible for our society? Better yet, perhaps we need to begin to realize the vision of Robert F. Kennedy:
There are those that look at things the way they are, and ask why? I dream of things that never were, and ask why not.
Things that never were? But they are already existing elsewhere!
Better yet, download
A Coherent Curriculum: The Case for Mathematics by William Schmidt, Richard Houang, and Leland Cogan is available online in American Educator, Summer 2002, pp. 1-17.
This was a call to action five years ago and we're still arguing about it today...
Posted by
Dave Marain
at
6:33 AM
0
comments
![]()
![]()
Labels: national math curriculum, national math curriculum england, national math panel, national standards
Saturday, March 3, 2007
Some updates...
1. I received a reply from Jennifer Graban of the National Math Panel. Her comments indicated recognition by the panel that there is a need for greater participation of classroom teachers, algebra teachers in particular. I decided to call Jennifer personally and we had a very pleasant conversation. Electronic communication and blogs can never replace human interaction! I'll share the email and our dialogue later...
2. I've posted a solution in the comment section to the last part of the nested radical problem -- finding an expression for N that would produce an integer value for the infinite nested radical.
3. You may want to read the comment I posted regarding Math Anxiety. It describes a method that I have found useful for conscientious students who tend to underperform on teacher-made tests. There is also a wonderful set of tips for students to prepare for standardized tests like SATs. It's entitled Ten Ways to Survive the Math Blues from Murray Bourne at squarecirclez and I will share this with the students in my school.
Posted by
Dave Marain
at
6:35 PM
0
comments
![]()
![]()
Labels: national math panel
Friday, March 2, 2007
An Online Pi Day Challenge
Even though pi day is about 2 weeks away, here's an online challenge I gave to all the students in my school back in 2005. Not very 'mathematical' but it engaged them. I posted the problem at 6 PM on March 14th and one student found a web site and submitted her solution in under 7 minutes! Get the feeling that this generation is able to learn a slightly different way!! For those offended by the fact that pi day is celebrated in other countries on July 22nd (22/7), I apologize! I am well aware that many teachers, math departments and schools have created wonderful pi day activities and there are abundant examples of these on the web. I would like others to share their favorites as well. Learning more about pi from MathWorld or Wikipedia is well worth our time, although it does get very technical. The nested radical problem from the other day (with the square roots of 2) relates to pi! Look it up!!
Background: The first 5 places (decimal digits) in pi, after the decimal point, are 14159. Find a web site that will allow you to search millions of places in pi.
The Challenge:
(1) Write down the seven decimal places in pi starting with the digit in the 5,191,306th place.
(2) These digits are a clue to the birthday of a famous mathematician (sorry, it's not Einstein!).
Give the full name of this mathematician and how he is connected to the number pi! Your submission should include the full birth date, the name and the connection.
EXTRA: Now challenge us - find another famous mathematician who is associated with pi and ask your own 'pi' challenge question!
Posted by
Dave Marain
at
6:00 AM
3
comments
![]()
![]()
Thursday, March 1, 2007
Is Math Anxiety Caused by More than Lack of Practice and Skill?
You may have to register first, but you might want to link to the article Understanding Math Anxiety in a recent edition of Teacher Magazine. I would be interested in the perspectives of both math classroom teachers and those outside of the profession. Many teachers I've spoken to feel that anxiety decreases with increased student preparation but from my own personal observations, I suspect there's more to it than that. Do you believe that 'performance anxiety' on standardized math tests is real or a fabrication? If real, what are the implications for the classroom teacher? Simply make our classes more rigorous and our tests harder so that students will be accustomed to performing under more pressure? Or is something else needed...
One of the cognitive psychologists quoted in the article, Robert Siegler, is a professor of cognitive psychology at Carnegie Mellon University. Note below his current participation on the National Math Panel. Here is a quote:
Students feel more anxiety in math partly because they are dealing with so many concepts and procedures that are foreign to them, said Robert S. Siegler, a professor of cognitive psychology at Carnegie Mellon University, in Pittsburgh, who has examined children’s thinking abilities in math and science. Once students realize they do not grasp a math concept, the internal pressure grows.“Math entails certain conceptual barriers that lead people to read the same passage over and over again and not understand it,” Mr. Siegler said. By contrast, in reading a history lesson, students are likely to recognize vocabulary, themes, and ideas, even if they do not understand all the implications of a particular passage.
“You don’t feel like you totally didn’t understand it, and you’re just floundering,” he said.
Mr. Siegler is one of 17 people serving on the National Mathematics Advisory Panel, a White House-commissioned group charged with identifying effective strategies for improving instruction in the subject. The panel includes a number of cognitive psychologists, along with education researchers, mathematicians, and others.
Posted by
Dave Marain
at
2:34 PM
2
comments
![]()
![]()
Labels: math anxiety, performance, pressure, standardized tests





